Embark on an enlightening journey with our comprehensive Unit 7 Exponential and Logarithmic Functions Answer Key. This definitive resource unveils the intricate world of exponential and logarithmic functions, empowering you with a profound understanding of their concepts, properties, and applications.

Delve into the fundamental principles of exponential functions, unraveling their unique characteristics and exploring real-world examples. Discover the inverse relationship between exponential and logarithmic functions, unlocking the secrets of converting between these forms effortlessly. Master the art of solving exponential equations using logarithmic functions, gaining invaluable problem-solving skills.

Exponential Functions

Exponential functions model exponential growth or decay, where the rate of change is proportional to the current value. They take the form f(x) = a ^x, where a is a positive constant called the base.

Examples:

• Population growth: f(t) = 1000(1.05) ^t, where t is the number of years
• Radioactive decay: f(t) = 100(0.5) ^t, where t is the number of years

Properties:

• f(0) = 1
• f(x) > 0 for all x
• f(x + y) = f(x)f(y)
• f(x – y) = f(x)/f(y)

Logarithmic Functions

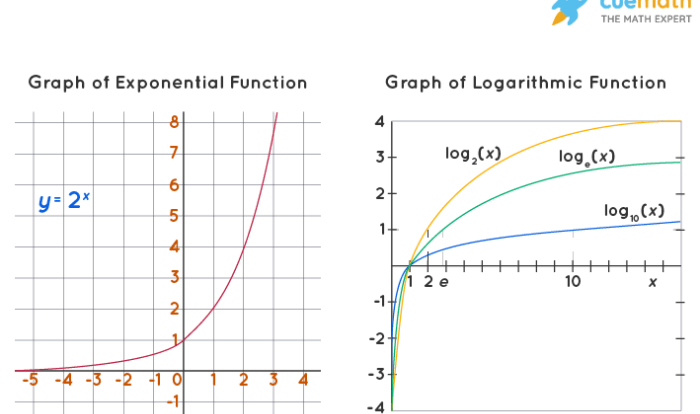
Logarithmic functions are the inverse of exponential functions. They take the form f(x) = log _ax, where a is a positive constant called the base. They model the inverse relationship between the base and the exponent.

Examples:

• The inverse of f(x) = 1000(1.05) ^tis g(x) = log _1.05x
• The inverse of f(x) = 100(0.5) ^tis g(x) = log _0.5x

Properties:

• f(a) = 1
• f(x) > 0 for x > 0
• f(xy) = f(x) + f(y)
• f(x/y) = f(x) – f(y)

Relationships between Exponential and Logarithmic Functions

Exponential and logarithmic functions are inverse functions. This means that if f(x) = a ^x, then g(x) = log _ax.

We can convert between exponential and logarithmic forms using the following equations:

• a ^log_ax = x
• log _aa ^x= x

Logarithmic functions can be used to solve exponential equations. For example, to solve the equation 2 ^x= 100, we can take the logarithm of both sides:

• log ₂2 ^x= log ₂100
• x = log ₂100

Applications of Exponential and Logarithmic Functions: Unit 7 Exponential And Logarithmic Functions Answer Key

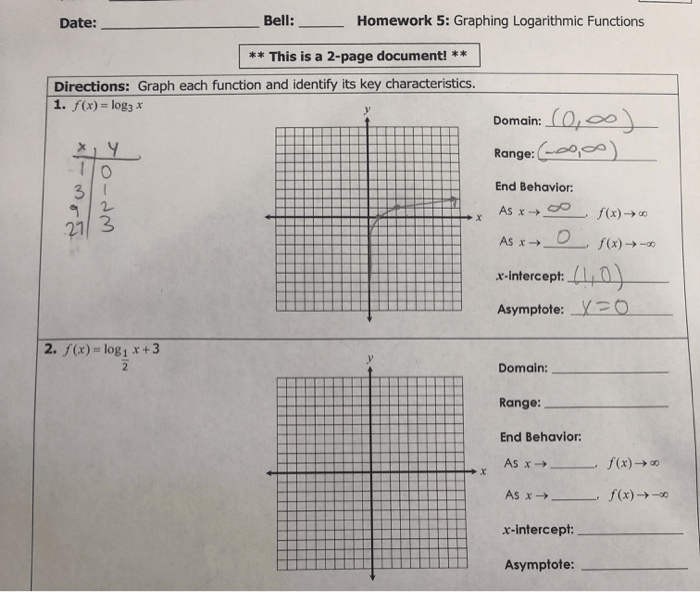
Exponential and logarithmic functions have a wide range of applications in various fields, including:

Exponential Functions:

• Modeling population growth and decay
• Describing radioactive decay
• Calculating compound interest

Logarithmic Functions:

• Measuring acidity (pH)
• Measuring sound intensity (decibels)
• Solving exponential equations

Commonly Asked Questions

What is the inverse function of an exponential function?

Logarithmic function

How do you convert an exponential equation to a logarithmic equation?

Take the logarithm of both sides of the equation

What are some real-world applications of exponential functions?

Modeling population growth, radioactive decay, and compound interest